| |
| |
Caption: Mathematical Models by Arnold Emch - Series 4 (1928)
This is a reduced-resolution page image for fast online browsing.
EXTRACTED TEXT FROM PAGE:
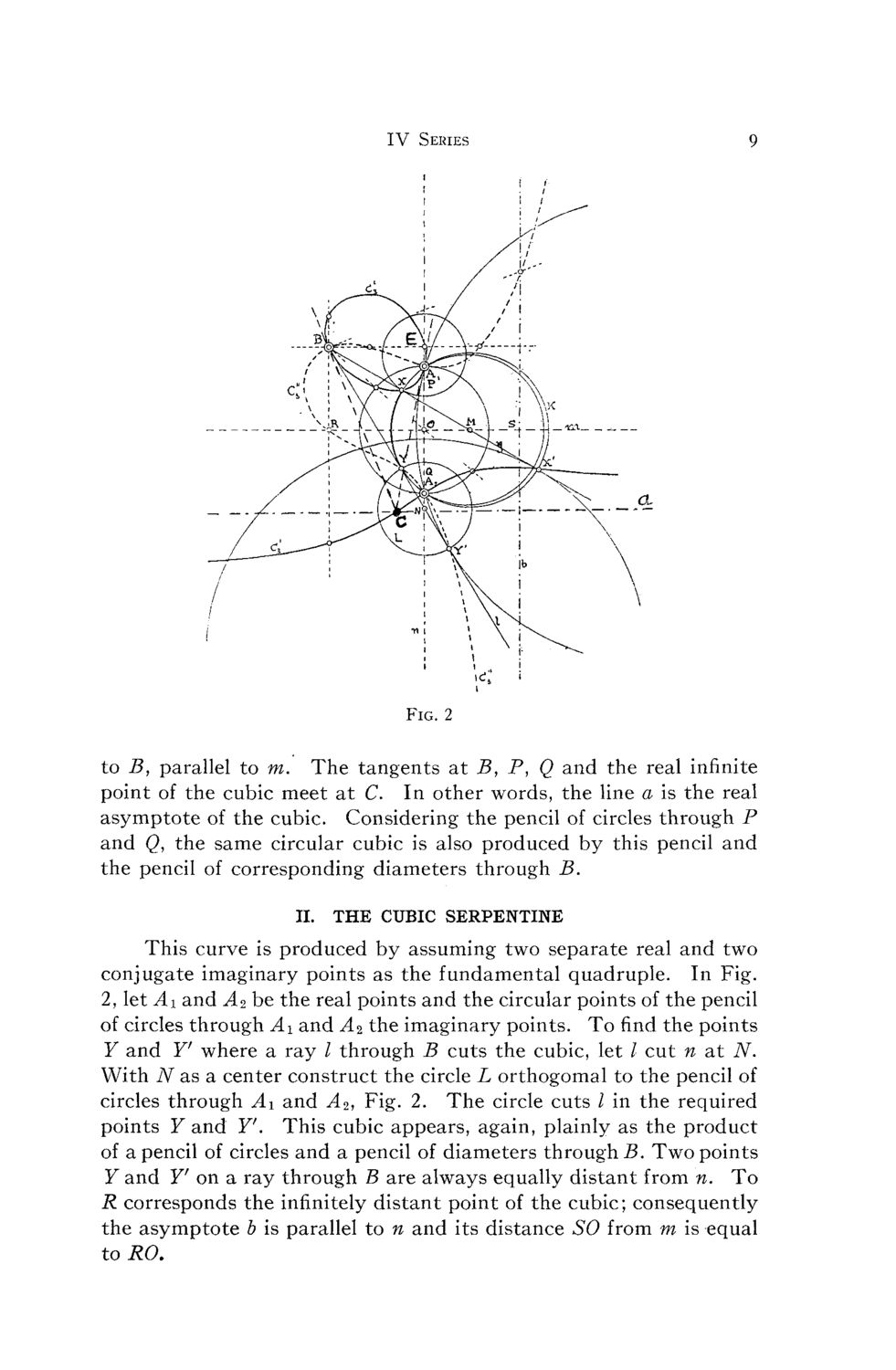
IV Series 9 i Fig. 2 to i?, parallel to m. The tangents at B, P, Q and the real infinite point of the cubic meet at C. In other words, the line a is the real asymptote of the cubic. Considering the pencil of circles through P and Q, the same circular cubic is also produced by this pencil and the pencil of corresponding diameters through B. II. THE CUBIC SERPENTINE This curve is produced by assuming two separate real and two conjugate imaginary points as the fundamental quadruple. In Fig. 2,\etAi and A2 be the real points and the circular points of the pencil of circles through Ai and A2 the imaginary points. T ofindthe points Y and Y' where a ray / through B cuts the cubic, let / cut n at N . With N as a center construct the circle L orthogomal to the pencil of circles through Ai and A2, Fig. 2. The circle cuts I in the required points Y and Y ' This cubic appears, again, plainly as the product '. of a pencil of circles and a pencil of diameters through B. T w o points Y and Y' on a ray through B are always equally distant from n. T o R corresponds the infinitely distant point of the cubic; consequently the asymptote b is parallel to n and its distance S O from m is equal to RO.
| |